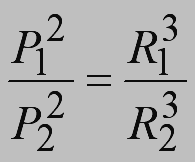
How fast do the planets move on their orbits?

We can calculate the relationship of the speed of one planet compared with the others, using Kepler's third law.

We start with the third law:

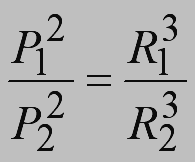
and rearrange it:

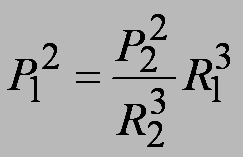
or

where C is a constant. Recall that the distance something travels is equal to its speed multiplied by the time it travels. Therefore, the speed is the distance divided by the time. The time for a planet to go around its orbit is, by definition, the period of its motion around the orbit. The distance our planet travels is

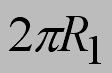
so its speed (dustance divided by time) is

That is, the speed goes inversely with the square root of the distance from the sun.

Here is the result. The relationship we just determined, speed goes inversely with the square root of the distance from the sun, is plotted as the line. The actual speeds of the planets fall right on that line, showing how closely they follow Kepler's third law. The earth is moving at about 30 km/sec,  or about 67,000 miles per hour! (from Bennett et al., The Essential Cosmic Perspective)