Yes, resolution is what the optometrist is checking when she has you read an eyechart.

We measure resolution in terms of the smallest angle, or direction of sight, we can perceive a change over. Angles are discussed in terms based on the old Babylonian base-60 number system. Thus, there are 360 degrees going around an entire circle. There are 60 minutes of arc, arcminutes, in a degree, and 60 seconds of arc, arcseconds, in an arcminute. The apparent size of the moon in the sky is about half a degree, or 30 arcminutes. The smallest details you can see with your eyes (not using a telescope to help) are a few arcminutes.

A more natural unit that is not based on the Babylonian numbering system is the radian. There are about 60 degrees in a radian. However, other than the example in the notes just before the link to this essay, we will stick with degrees, arcminutes, and arcseconds in the course.

As with your eyes, the resolution can be made worse if the lenses or other optics of a telescope are not perfectly shaped. However, even if the optics are perfect, there is a limit to the resolution - the smallest line you could read on the eyechart. For astronomers, the larger the telescope the better resolution it can provide. Here is why.

This result can be explained in terms of Young's fringes discussed earlier:

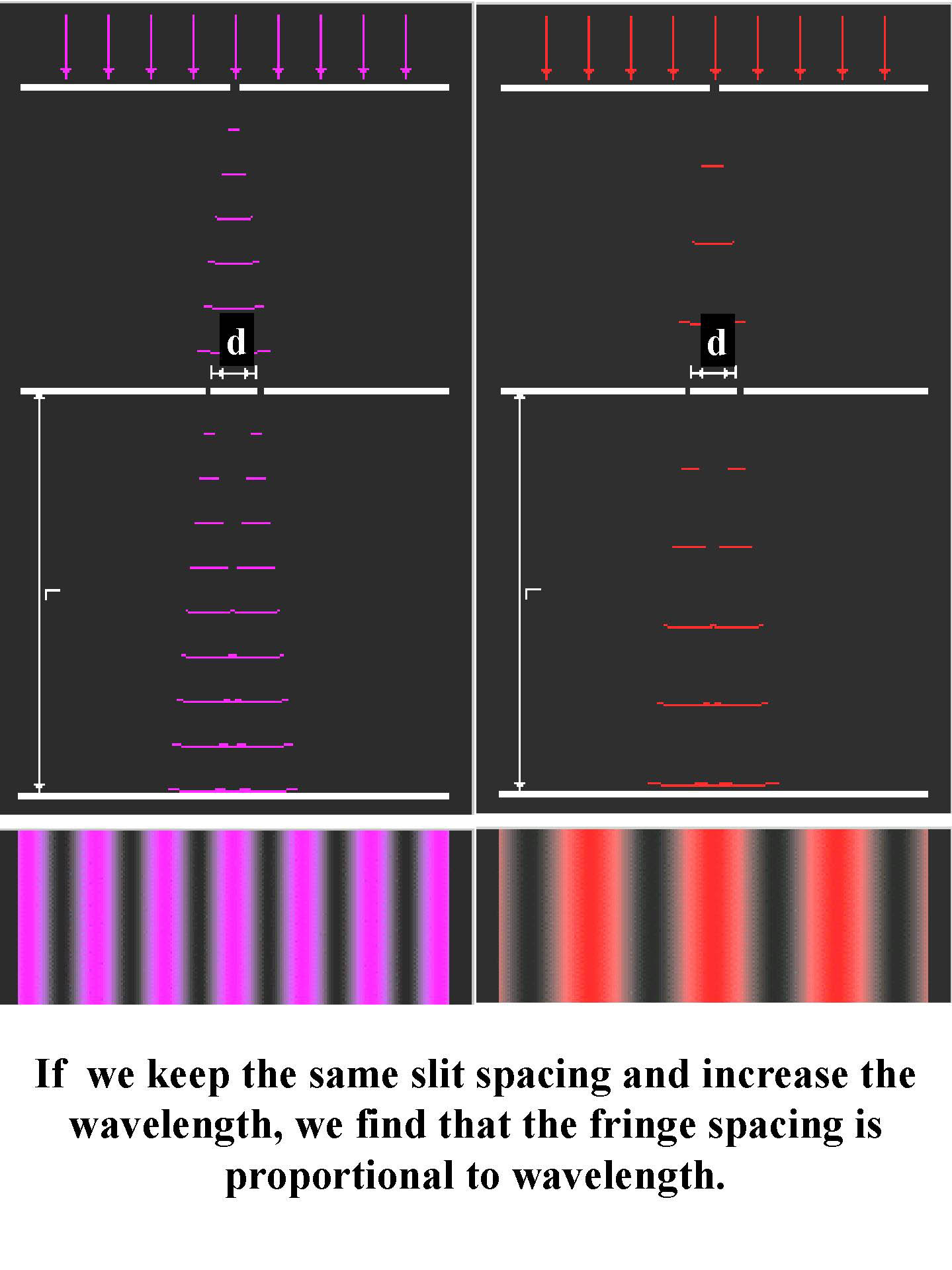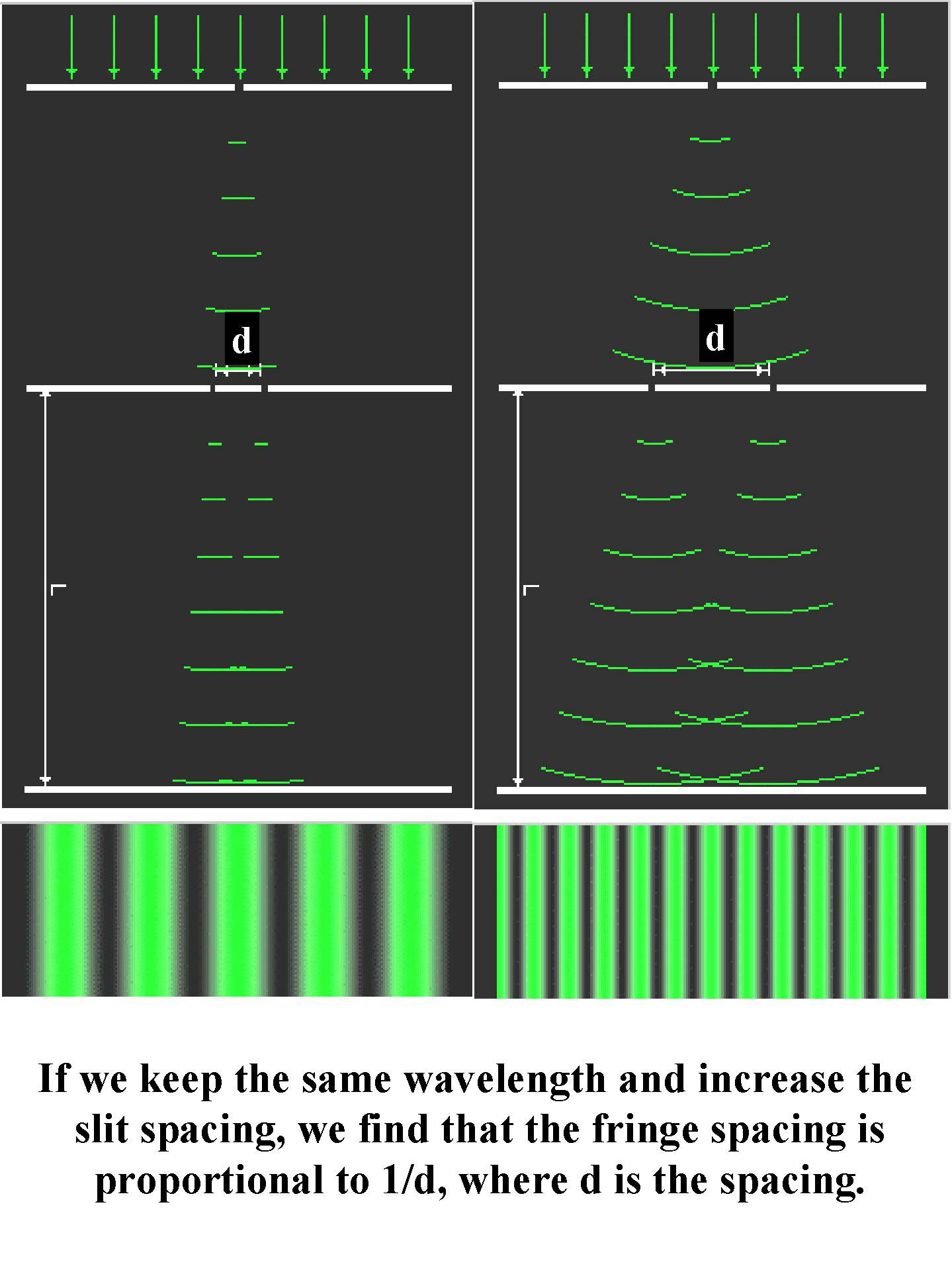To experiment, try this link:  http://www.colorado.edu/physics/2000/schroedinger/two-slit2.html

In fact, the fringe spacing is just proportional to the distance between the slits measured in wavelengths of the light, or to /d. We can do a similar experiment by putting two telescopes of the "Very Large Array" (near Socorro, New Mexico) where the slits are - the VLA is just like a giant, multiple Young's fringes device. 

With lots of telescopes, only the fringe in the center is reinforced by all the spacings, and the others tend to cancel - giving us the principle of an imaging interferometer like the VLA.:

(Picture by D. Finley, http://www.aoc.nrao.edu/intro/vlapix/vlaviews.index.html)

The more telescopes, the  better the fringes cancel - until we get a filled mirror like an optical telescope. The resolution will still go as /D (where D is now the maximum distance between telescopes in an interferometer, or the diameter of a telescope mirror), but the images will be cleaner.